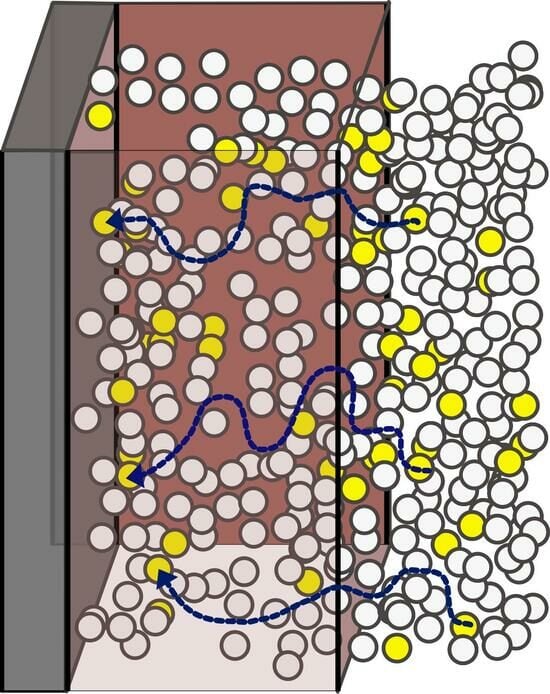
Contamination and Decontamination of Polymer-Coated Surfaces
Abstract
:1. Introduction
2. Classical Density Functional Theory as a Tool for Calculating the Potential of Mean Force
3. The Model System and Computational Details
4. Results for the Equilibrium System
4.1. Potentials of Mean Force Experienced by the Contaminant
4.2. Equilibrium Structure inside Grafted Polymer Layers
5. Effect of the Potential of Mean Force on the Molecular Diffusion inside Grafted Polymer Layers
5.1. Contaminant Penetration through a Grafted Polymer Layer
5.2. Diffusion-Driven Surface Decontamination
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Einstein, A. Über die von der molekularkinetischen Theorie der Wärme geforderte Bewegung von in ruhenden Flüssigkeiten suspendierten Teilchen. Ann. Phys. 1905, 17, 549–560. [Google Scholar] [CrossRef]
- von Smoluchowski, M. Über Brownsche Molekularbewegung unter Einwirkung äußerer Kräfte und deren Zusammenhang mit der verallgemeinerten Diffusionsgleichung. Ann. Phys. 1915, 353, 1103–1112. [Google Scholar] [CrossRef]
- van Swol, F.; Frink, L.J.D.; Malanoski, A.P.; Petsev, D.N. Diffusion in Inhomogeneous Fluids: Hard Spheres to Polymer Coatings. J. Chem. Phys. 2023, 159, 084705. [Google Scholar] [CrossRef]
- Marconi, U.M.B.; Tarazona, P. Dynamic density functional theory of fluids. J. Chem. Phys. 1999, 110, 8032–8044. [Google Scholar] [CrossRef]
- Archer, A.J.; Evans, R. Dynamical density functional theory and its application to spinodal decomposition. J. Chem. Phys. 2004, 121, 4246–4254. [Google Scholar] [CrossRef] [PubMed]
- Archer, A.J. Dynamical density functional theory: Binary phase-separating colloidal fluid in a cavity. J. Phys. Condens. Matter 2005, 17, 1405–1427. [Google Scholar] [CrossRef]
- Piazza, F.; Dorsaz, N.; Michele, C.D.; Rios, P.D.L.; Foffi, G. Diffusion-limited reactions in crowded environments: A local density approximation. J. Phys. Condens. Matter 2021, 25, 375104. [Google Scholar] [CrossRef]
- te Vrugt, M.; Löwen, H.; Wittkowski, R. Classical dynamical density functional theory: From fundamentals to applications. Adv. Phys. 2020, 69, 121–247. [Google Scholar] [CrossRef]
- Fleer, G.; Stuart, M.C.; Scheutjens, J.; Cosgrove, T.; Vincent, B. Polymers at Interfaces; Springer: New York, NY, USA, 1993. [Google Scholar]
- Carignano, M.A.; Szleifer, I. Statistical thermodynamic theory of grafted polymeric layers. J. Chem. Phys. 1993, 98, 5006–5018. [Google Scholar] [CrossRef]
- Szleifer, I.; Carignano, M.A. Tethered polymer layers. Adv. Chem. Phys. 1996, 94, 165–260. [Google Scholar]
- Fredrickson, G. The Equilibrium Theory of Inhomogeneous Polymers; Oxford University Press: New York, NY, USA, 2005. [Google Scholar]
- Jain, S.; Dominik, A.; Chapman, W.G. Modified interfacial statisical associating fluid theory: A perturbation density functional theory for inhomogeneous complex fluids. J. Chem. Phys. 2007, 127, 244904. [Google Scholar] [CrossRef] [PubMed]
- Wertheim, M.S. Fluids with highly directional attractive forces. I. Statistical thermodynamics. J. Stat. Phys. 1984, 35, 19–34. [Google Scholar] [CrossRef]
- Jain, S.; Jog, P.; Weinhold, J.; Srivastava, R.; Chapman, W. Modified interfacial statistical associating fluid theory: Application to tethered polymer chains. J. Phys. Condens. Matter 2008, 128, 154910. [Google Scholar] [CrossRef] [PubMed]
- Rosenfeld, Y. Scaled Field Particle Theory of the Structure and the Thermodynamics of Isotropic Hard Particle Fluids. J. Chem. Phys. 1988, 89, 4272–4287. [Google Scholar] [CrossRef]
- Rosenfeld, Y. Free–energy model for the inhomogeneous hard–sphere fluid mixture and density functional theory of freezing. Phys. Rev. Lett. 1989, 63, 980–983. [Google Scholar] [CrossRef] [PubMed]
- Roth, R. Fundamental measure theory for hard-sphere mixtures: A review. J. Phys. Condens. Matter 2010, 22, 063102. [Google Scholar] [CrossRef]
- Roth, R.; Evans, R.; Lang, A.; Kahl, G. Fundamental measure theory for hard-sphere mixtures revisited: The White Bear version. J. Phys. Cond. Matter 2002, 14, 12063–12078. [Google Scholar] [CrossRef]
- Rosenfeld, Y.; Schmidt, M.; Löwen, H.; Tarazona, P. Fundamental-measure free-energy density functional for hard spheres: Dimensional crossover and freezing. Phys. Rev. E 1997, 55, 4245–4263. [Google Scholar] [CrossRef]
- The Tramonto Project. Available online: https://tramonto.github.io (accessed on 29 October 2023).
- Frink, L.J.D.; Salinger, A.G. Two and three dimensional nonlocal density functional theory for inhomogeneous fluids I. Algorithms and Parallelization. J. Comp. Phys. 2000, 159, 407–424. [Google Scholar] [CrossRef]
- Frink, L.; Salinger, A.; Sears, M.; Weinhold, J.; Frishknecht, A. Numerical challenges in the application of density functional theory to biology and nanotechnology. J. Phys. Cond. Matter 2002, 14, 12167–12187. [Google Scholar] [CrossRef]
- Heroux, M.; Salinger, A.; Frink, L. Parallel Segregated Schur Complement Methods for Fluid Density Functional Theories. SIAM J. Sci. Comput. 2007, 29, 2059–2077. [Google Scholar] [CrossRef]











Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frink, L.J.D.; van Swol, F.; Serrano, A.; Petsev, D.N. Contamination and Decontamination of Polymer-Coated Surfaces. Colloids Interfaces 2023, 7, 69. https://doi.org/10.3390/colloids7040069
Frink LJD, van Swol F, Serrano A, Petsev DN. Contamination and Decontamination of Polymer-Coated Surfaces. Colloids and Interfaces. 2023; 7(4):69. https://doi.org/10.3390/colloids7040069
Chicago/Turabian StyleFrink, Laura J. D., Frank van Swol, Arianna Serrano, and Dimiter N. Petsev. 2023. "Contamination and Decontamination of Polymer-Coated Surfaces" Colloids and Interfaces 7, no. 4: 69. https://doi.org/10.3390/colloids7040069