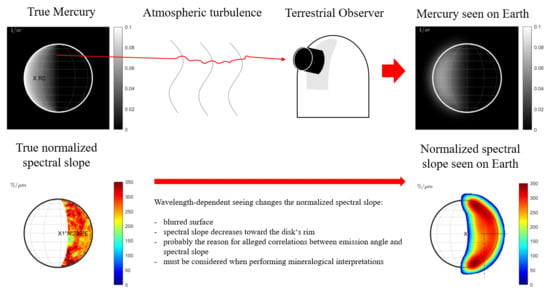
Wavelength-Dependent Seeing Systematically Changes the Normalized Slope of Telescopic Reflectance Spectra of Mercury
Abstract
:1. Introduction
2. Materials and Methods
2.1. Reflectance Modeling
2.2. Seeing Model
2.3. Modeling Various Observation Conditions
3. Results
3.1. Simulation of Ideal Scenario
3.2. Simulation of Previous Measurement Campaigns
3.2.1. Systematic Effects of the Normalized Spectral Slopes
3.2.2. Slope Differences between Campaigns
4. Discussion and Conclusion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| MESSENGER | Mercury Surface, Space Environment, Geochemistry, and Ranging |
| NIR | Near-infrared |
| TIR | Thermal infrared |
| MDIS | Mercury Dual Imaging System |
| MASCS | Mercury Atmospheric and Surface Composition Spectrometer |
| FWHM | Full width half maximum |
| SVST | Swedish Vacuum Solar Telescope |
| NOT | Nordic Optical Telescope |
| ALFOCS | Alhambra Faint Object Spectrograph and Camera |
| IRTF | Infrared Telescope Facility |
| NTT | New Technology Telescope |
| Sofi | Son of ISAAC |
| SELO | Sub-Earth longitude |
| SELA | Sub-earth latitude |
| SSLO | Sub-Solar longitude |
| SSLA | Sub-Solar latitude is approximately zero and not displayed |
| AD | Angular diameter |
| DHG | Double Henyey–Greenstein |
| KS | Kaasalainen–Shkuratov (model) |
| KS3 | Kaasalainen–Shkuratov (model) version 3 |
| PSF | Point spread function |
References
- McCord, T.B.; Adams, J.B. Mercury: Surface Composition from the Reflection Spectrum. Science 1972, 178, 745–747. [Google Scholar] [CrossRef]
- Vilas, F.; McCord, T.B. Mercury: Spectral reflectance measurements (0.33–1.06 mum) 1974/1975. Icarus 1976, 28, 593–599. [Google Scholar] [CrossRef]
- Sprague, A.L.; Emery, J.P.; Donaldson, K.L.; Russel, R.W.; Lynch, D.K.; Mazuk, A.L. Mercury: Mid-infrared (3–13.5 mum) observations show heterogeneous composition, presence of intermediate and basic soil types, and pyroxene. Meteorit. Planet. Sci. 2002, 37, 1255–1268. [Google Scholar] [CrossRef]
- Warell, J.; Limaye, S. Properties of the Hermean regolith: I. Global regolith albedo variation at 200km scale from multicolor CCD imaging. Planet. Space Sci. 2001, 49, 1531–1552. [Google Scholar] [CrossRef]
- Warell, J. Properties of the Hermean Regolith: II. Disk-Resolved Multicolor Photometry and Color Variations of the “Unknown” Hemisphere. Icarus 2002, 156, 303–317. [Google Scholar] [CrossRef]
- Warell, J. Properties of the hermean regolith: III. disk-resolved vis–NIR reflectance spectra and implications for the abundance of iron—Based on observations made with the Nordic Optical Telescope, operated on the island of La Palma jointly by Denmark, Finland, Iceland, Norway, and Sweden, in the Spanish Observatorio del Roque de los Muchachos of the Instituto de Astrofisica de Canarias. Icarus 2003, 161, 199–222. [Google Scholar] [CrossRef]
- Warell, J. Properties of the Hermean regolith: IV. Photometric parameters of Mercury and the Moon contrasted with Hapke modelling. Icarus 2004, 167, 271–286. [Google Scholar] [CrossRef]
- Warell, J.; Blewett, D. Properties of the Hermean regolith: V. New optical reflectance spectra, comparison with lunar anorthosites, and mineralogical modelling. Icarus 2004, 168, 257–276. [Google Scholar] [CrossRef]
- Warell, J.; Sprague, A.; Emery, J.; Kozlowski, R.; Long, A. The 0.7–5.3 mum IR spectra of Mercury and the Moon: Evidence for high-Ca clinopyroxene on Mercury. Icarus 2006, 180, 281–291. [Google Scholar] [CrossRef]
- Vernazza, P.; DeMeo, F.; Nedelcu, D.; Birlan, M.; Doressoundiram, A.; Erard, S.; Volquardsen, E. Resolved spectroscopy of Mercury in the near-IR with SpeX/IRTF. Icarus 2010, 209, 125–137. [Google Scholar] [CrossRef]
- Erard, S.; Bézard, B.; Doressoundiram, A.; Despan, D. Mercury resolved spectroscopy from NTT. Planet. Space Sci. 2011, 59, 1842–1852. [Google Scholar] [CrossRef]
- Murchie, S.L.; Klima, R.L.; Domingue, D.L.; Izenberg, N.R.; Blewett, D.T.; Helbert, J. Spectral reflectance constraints on the composition and evolution of Mercury’s surface. In Mercury: The view after MESSENGER; Solomon, S.C., Nittler, L.R., Anderson, B.J., Eds.; Cambridge University Press: Cambridge, UK, 2019. [Google Scholar]
- Varatharajan, I.; Tsang, C.; Wohlfarth, K.; Wöhler, C.; Izenberg, N.; Helbert, J. Surface Composition of Mercury from NIR (0.7–4.2 μm) Ground-Based IRTF/SpeX Spectroscopy. European Planetay Science Congress 2019, Abstract No. 1331. 2019. Available online: https://meetingorganizer.copernicus.org/EPSC-DPS2019/EPSC-DPS2019-1331-2.pdf (accessed on 7 December 2021).
- Hawkins, S.E.I.; Boldt, J.; Darlington, E.; Espiritu, R.; Gold, R.; Gotwols, B.; Grey, M.; Hash, C.; Hayes, J.; Jaskulek, S.; et al. The mercury dual imaging system on tFhe MESSENGER spacecraft. Space Sci. Rev. 2007, 131, 247–338. [Google Scholar] [CrossRef]
- Hawkins, S.E.I.; Murchie, S.; Becker, K.; Selby, C.; Turner, F.; Noble, M.; Chabot, N.; Choo, T.; Darlington, E.; Denevi, B.; et al. In-Flight performance of MESSENGER’s Mercury dual imaging system. In Proceedings of the SPIE—The International Society for Optical Engineering, San Diego, CA, USA, 3–4 August 2009; Volume 7441. [Google Scholar] [CrossRef]
- McClintock, W.; Lankton, M. The Mercury Atmospheric and Surface Composition Spectrometer for the MESSENGER mission. Space Sci. Rev. 2007, 131, 481–521. [Google Scholar] [CrossRef]
- Domingue, D.L.; Denevi, B.W.; Murchie, S.L.; Hash, C.D. Application of multiple photometric models to disk-resolved measurements of Mercury’s surface: Insights into Mercury’s regolith characteristics. Icarus 2016, 268, 172–203. [Google Scholar] [CrossRef] [Green Version]
- Boyd, R.W. The wavelength dependence of seeing. J. Opt. Soc. Am. 1978, 68, 877–883. [Google Scholar] [CrossRef] [Green Version]
- Hapke, B. Bidirectional Reflectance Spectroscopy: 5. The Coherent Backscatter Opposition Effect and Anisotropic Scattering. Icarus 2002, 157, 523–534. [Google Scholar] [CrossRef] [Green Version]
- Hapke, B. Theory of Reflectance and Emittance Spectroscopy, 2nd ed.; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Vilas, F.; Leake, M.A.; Mendell, W.W. The dependence of reflectance spectra of Mercury on surface terrain. Icarus 1984, 59, 60–68. [Google Scholar] [CrossRef]
- Warell, J.; Davidsson, B. A Hapke model implementation for compositional analysis of VNIR spectra of Mercury. Icarus 2010, 209, 164–178. [Google Scholar] [CrossRef]
- Shepard, M.K.; Helfenstein, P. A test of the Hapke photometric model. J. Geophys. Res. Planets 2007, 112, E03001. [Google Scholar] [CrossRef] [Green Version]
- Shkuratov, Y.; Kaydash, V.; Korokhin, V.; Velikodsky, Y.; Petrov, D.; Zubko, E.; Stankevich, D.; Videen, G. A critical assessment of the Hapke photometric model. J. Quant. Spectrosc. Radiat. Transf. 2012, 113, 2431–2456. [Google Scholar] [CrossRef]
- Shkuratov, Y.; Kaydash, V.; Korokhin, V.; Velikodsky, Y.; Opanasenko, N.; Videen, G. Optical measurements of the Moon as a tool to study its surface. Planet. Space Sci. 2011, 59, 1326–1371. [Google Scholar] [CrossRef]
- Moffat, A.F.J. A Theoretical Investigation of Focal Stellar Images in the Photographic Emulsion and Application to Photographic Photometry. Astron. Astrophys. 1969, 3, 455. [Google Scholar]
- Becker, K.J.; Robinson, M.S.; Becker, T.L.; Weller, L.A.; Turner, S.; Nguyen, L.; Selby, C.; Denevi, B.W.; Murchie, S.L.; McNutt, R.L.; et al. Near Global Mosaic of Mercury. In AGU Fall Meeting Abstracts; American Geophysical Union: San Francisco, CA, USA, 2009; Volume 2009, p. P21A-1189. [Google Scholar]
- Marsset, M.; DeMeo, F.E.; Binzel, R.P.; Bus, S.J.; Burbine, T.H.; Burt, B.; Moskovitz, N.; Polishook, D.; Rivkin, A.S.; Slivan, S.M.; et al. Twenty Years of SpeX: Accuracy Limits of Spectral Slope Measurements in Asteroid Spectroscopy. Astrophys. J. Suppl. Ser. 2020, 247, 73. [Google Scholar] [CrossRef] [Green Version]
















| Campaign | No | Date | Time UTC | SELO | SELA | SSLO | AD | S | |
|---|---|---|---|---|---|---|---|---|---|
| Warell and Limaye [4] | 01 | 20 October 1995 | 08:00 | 273.71 | 2.68 | 359.43 | 85.7 | 6.94 | |
| SVST | 02 | 22 October 1995 | 07:30 | 283.74 | 2.32 | 359.64 | 75.9 | 6.57 | |
| 550–940 nm | 03 | 19 April 1996 | 15:00 | 91.43 | −2.35 | 2.01 | 89.4 | 7.09 | |
| 04 | 22 November 1997 | 17:30 | 213.22 | −1.27 | 154.58 | 58.6 | 5.92 | ||
| 05 | 24 November 1997 | 16:30 | 222.82 | −1.53 | 159.42 | 63.4 | 6.12 | ||
| 06 | 9 July 1998 | 19:00 | 307.68 | 6.34 | 223.69 | 84 | 6.98 | ||
| 07 | 27 April 1999 | 07:30 | 58.35 | −1.41 | 143 | 75.6 | 6.59 | ||
| Warell [6] | 08 | 20 June 1999 | 20:41–21:02 | 291.8 | 4.8 | 207 | 84.8 | 7.0 | |
| NOT with ALFOCS | 09 | 22 June 1999 | 20:23–20:35 | 301.5 | 5.2 | 212.5 | 89.1 | 7.2 | |
| 520–970 nm | |||||||||
| Warell and Blewett [8] | 10 | 1 July 2002 | 05:57 | 277.1 | 5.3 | 354.7 | 60.7 | 6.5 | |
| NOT with ALFOCS | |||||||||
| 400–670 nm | |||||||||
| Warell et al. [9] | 11 | 26 June 2002 | 17:48–19:47 | 241 | 5.6 | 341 | 99.4 | 7.7 | 1 |
| IRTF with SpeX | 12 | 17 August 2003 | 18:06–21:41 | 197 | 8.2 | 102 | 95.3 | 7.8 | 1 |
| 700–5300 nm | 13 | 17 August 2003 | 18:22–21:48 | 197 | 8.2 | 102 | 95.3 | 7.8 | 1 |
| Vernazza et al. [10] | 14 | 28 February 2008 | 19:30–20:30 | 141 | −7 | 27 | 89 | 7.5 | 1.5 |
| IRTF with SpeX | 15 | 29 February 2008 | 18:45–20:30 | 147 | −7 | 27 | 87 | 7.4 | 1.5 |
| 900–2400 nm | 16 | 22 February 2008 | 18:45–20:30 | 254 | −4 | 21 | 53 | 5.6 | 1 |
| 17 | 23 March 2008 | 18:30–20:30 | 258 | −4 | 21 | 51 | 5.6 | 2 | |
| 18 | 13 May 2008 | 21:00–23:30 | 120 | 1 | 22 | 105 | 8.0 | 2 | |
| 19 | 14 May 2008 | 21:00–23:00 | 125 | 1 | 22 | 107 | 8.2 | 1.5 | |
| Erard et al. [11] | 20 | 16 June 2006 | 22:20–22:32 | 233.24 | 4.82 | 327.7 | 99.44 | 7.5 | 1.6 |
| NTT with Sofi | |||||||||
| 940–2500 nm | |||||||||
| Varatharajan et al. [13] | 21 | 16 December 2018 | 20:15 | 58.51 | −3.92 | 348.06 | 70.50 | 6.4 | |
| IRTF with SpeX | |||||||||
| 700–2400 nm |
| Parameter | Description | F (430 nm) | G (750 nm) | I (1000 nm) |
|---|---|---|---|---|
| b | DHG-function; asymmetry | 0.1551 | 0.1223 | 0.1339 |
| c | DHG-function; | 0.7478 | 0.8542 | 0.6642 |
| roughness | 14.6013° | 14.2707° | 14.0050° |
| Parameter | Description | F (430 nm) | G (750 nm) | I (1000 nm) |
|---|---|---|---|---|
| a | Phase function exponent | 0.6363 | 0.5628 | 0.5200 |
| Disk function weight | 0.6293 | 0.6424 | 0.6303 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wohlfarth, K.; Wöhler, C. Wavelength-Dependent Seeing Systematically Changes the Normalized Slope of Telescopic Reflectance Spectra of Mercury. Remote Sens. 2022, 14, 405. https://doi.org/10.3390/rs14020405
Wohlfarth K, Wöhler C. Wavelength-Dependent Seeing Systematically Changes the Normalized Slope of Telescopic Reflectance Spectra of Mercury. Remote Sensing. 2022; 14(2):405. https://doi.org/10.3390/rs14020405
Chicago/Turabian StyleWohlfarth, Kay, and Christian Wöhler. 2022. "Wavelength-Dependent Seeing Systematically Changes the Normalized Slope of Telescopic Reflectance Spectra of Mercury" Remote Sensing 14, no. 2: 405. https://doi.org/10.3390/rs14020405