Micromechanical Modeling of the Biaxial Deformation-Induced Phase Transformation in Polyethylene Terephthalate
Abstract
:1. Introduction
2. Model
2.1. Micromechanics-Based Theory for Deformation-Induced Phase Transformation
2.2. Model Implementation
3. Results and Discussion
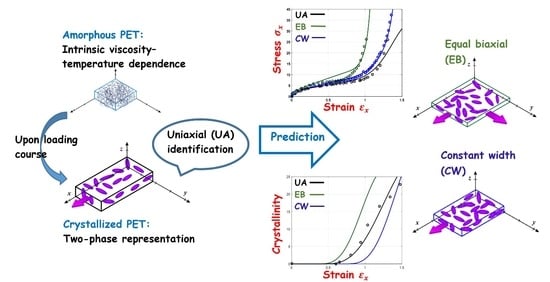
3.1. Model Identification
3.1.1. Continuous Amorphous Phase Properties
3.1.2. Discrete Crystalline Phase Properties
3.1.3. Overall Response
3.2. Model Prediction
3.2.1. Comparison between Model and Experiments
3.2.2. Implication of the Phase Changes
4. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Buckley, C.P.; Jones, D.C.; Jones, D.P. Hot-drawing of poly(ethylene terephthalate) under biaxial stress: Application of a three-dimensional glass-rubber constitutive model. Polymer 1996, 37, 2403–2414. [Google Scholar]
- Adams, A.M.; Buckley, C.P.; Jones, D.P. Biaxial hot drawing of poly(ethylene terephthalate): Measurements and modelling of strain-stiffening. Polymer 2000, 41, 771–786. [Google Scholar]
- Boyce, M.C.; Socrate, S.; Llana, P.G. Constitutive model for the finite deformation stress-strain behavior of poly(ethylene terephthalate) above the glass transition. Polymer 2000, 41, 2183–2201. [Google Scholar]
- Doufas, A.K.; McHugh, A.J.; Miller, C. Simulation of melt spinning including flow-induced crystallization: Part I. Model development and predictions. J. Non-Newton. Fluid Mech. 2000, 92, 27–66. [Google Scholar]
- Ahzi, S.; Makradi, A.; Gregory, R.V.; Edie, D.D. Modeling of deformation behavior and strain-induced crystallization in poly(ethylene terephthalate) above the glass transition temperature. Mech. Mater. 2003, 35, 1139–1148. [Google Scholar]
- Makradi, A.; Ahzi, S.; Gregory, R.V.; Edie, D.D. A two-phase self-consistent model for the deformation and phase transformation behavior of polymers above the glass transition temperature: Application to PET. Int. J. Plast. 2005, 21, 741–758. [Google Scholar]
- Dupaix, R.B.; Krishnan, D. A constitutive model for strain-induced crystallization in poly(ethylene terephthalate) (PET) during finite strain load-hold simulations. J. Eng. Mater. Technol. 2006, 128, 28–33. [Google Scholar]
- Dupaix, R.B.; Boyce, M.C. Constitutive modeling of the finite strain behavior of amorphous polymers in and above the glass transition. Mech. Mater. 2007, 39, 39–52. [Google Scholar]
- Figiel, L.; Buckley, C.P. On the modelling of highly elastic flows of amorphous thermoplastics. Int. J. Non-Linear Mech. 2009, 44, 389–395. [Google Scholar]
- Chevalier, L.; Luo, Y.M.; Monteiro, E.; Menary, G.H. On visco-elastic modelling of polyethylene terephthalate behaviour during multiaxial elongations slightly over the glass transition temperature. Mech. Mater. 2012, 52, 103–116. [Google Scholar]
- Cosson, B.; Chevalier, L.; Régnier, G. Simulation of the stretch blow moulding process: From the modelling of the microstructure evolution to the end-use elastic properties of polyethylene terephthalate bottles. Int. J. Mater. Form. 2012, 5, 39–53. [Google Scholar]
- Menary, G.H.; Tan, C.W.; Harkin-Jones, E.M.A.; Armstrong, C.G.; Martin, P.J. Biaxial deformation and experimental study of PET at conditions applicable to stretch blow molding. Polym. Eng. Sci. 2012, 52, 671–688. [Google Scholar]
- Mahjoubi, H.; Zaïri, F.; Tourki, Z. A micro-macro constitutive model for strain-induced molecular ordering in biopolymers: Application to polylactide over a wide range of temperatures. Int. J. Plast. 2019, 123, 38–55. [Google Scholar]
- Mahjoubi, H.; Zaïri, F.; Tourki, Z. Strain-induced phase transformation in poly(lacticacid) across the glass transition: Constitutive model and identification. Int. J. Non-Linear Mech. 2020, 118, 103241. [Google Scholar]
- Ayoub, G.; Zaïri, F.; Naït-Abdelaziz, M.; Gloaguen, J.M. Modelling large deformation behaviour under loading-unloading of semicrystalline polymers: Application to a high density polyethylene. Int. J. Plast. 2010, 26, 329–347. [Google Scholar]
- Ayoub, G.; Zaïri, F.; Fréderix, C.; Gloaguen, J.M.; Naït-Abdelaziz, M.; Seguela, R.; Lefebvre, J.M. Effects of crystal content on the mechanical behaviour of polyethylene under finite strains: Experiments and constitutive modelling. Int. J. Plast. 2011, 27, 492–511. [Google Scholar]
- Abdul-Hameed, H.; Messager, T.; Zaïri, F.; Naït-Abdelaziz, M. Large-strain viscoelastic-viscoplastic constitutive modeling of semi-crystalline polymers and model identification by deterministic/evolutionary approach. Comput. Mater. Sci. 2014, 90, 241–252. [Google Scholar]
- Makki, M.; Ayoub, G.; Abdul-Hameed, H.; Zaïri, F.; Mansoor, B.; Naït-Abdelaziz, M.; Ouederni, M.; Zaïri, F. Mullins effect in polyethylene and its dependency on crystal content: A network alteration model. J. Mech. Behav. Biomed. Mater. 2017, 75, 442–454. [Google Scholar]
- Bernard, C.A.; Lame, O.; Deplancke, T.; Cavaillé, J.Y.; Ogawa, K. From rheological to original three-dimensional mechanical modelling of semi-crystalline polymers: Application to a wide strain rate range and large deformation of Ultra-High Molecular Weight PolyEthylene. Mech. Mater. 2020, 151, 103640. [Google Scholar]
- Lee, B.J.; Parks, D.M.; Ahzi, S. Micromechanical modeling of large plastic deformation and texture evolution in semi-crystalline polymers. J. Mech. Phys. Solids 1993, 41, 651–687. [Google Scholar]
- Lee, B.J.; Argon, A.S.; Parks, D.M.; Ahzi, S.; Bartczak, Z. Simulation of large strain plastic deformation and texture evolution in high density polyethylene. Polymer 1993, 34, 3555–3575. [Google Scholar]
- Nikolov, S.; Doghri, I.; Pierard, O.; Zealouk, L.; Goldberg, A. Multi-scale constitutive modeling of the small deformations of semi-crystalline polymers. J. Mech. Phys. Solids 2002, 50, 2275–2302. [Google Scholar]
- Van Dommelen, J.A.W.; Parks, D.M.; Boyce, M.C.; Brekelmans, W.A.M.; Baaijens, F.P.T. Micromechanical modeling of the elasto-viscoplastic behavior of semi-crystalline polymers. J. Mech. Phys. Solids 2003, 51, 519–541. [Google Scholar]
- Agoras, M.; Ponte Castaneda, P. Multi-scale homogenization-based modeling of semi-crystalline polymers. Philos. Mag. 2012, 92, 925–958. [Google Scholar]
- Poluektov, M.; van Dommelen, J.A.W.; Govaert, L.E.; MacKerron, D.H.; Geers, M.G.D. Micromechanical modeling of roll-to-roll processing of oriented polyethylene terephthalate films. J. Appl. Polym. Sci. 2016, 133, 43384. [Google Scholar]
- Bedoui, F.; Diani, J.; Régnier, G. Micromechanical modeling of elastic properties in polyolefins. Polymer 2004, 45, 2433–2442. [Google Scholar]
- Sedighiamiri, A.; Van Erp, T.B.; Peters, G.W.M.; Govaert, L.E.; van Dommelen, J.A.W. Micromechanical modeling of the elastic properties of semicrystalline polymers: A three-phase approach. J. Polym. Sci. Part B Polym. Phys. 2010, 48, 2173–2184. [Google Scholar]
- Bedoui, F.; Diani, J.; Régnier, G.; Seiler, W. Micromechanical modeling of isotropic elastic behavior of semicrystalline polymers. Acta Mater. 2006, 54, 1513–1523. [Google Scholar]
- Guan, X.; Pitchumani, R. A micromechanical model for the elastic properties of semicrystalline thermoplastic polymers. Polym. Eng. Sci. 2004, 44, 433–451. [Google Scholar]
- Ahzi, S.; Bahlouli, N.; Makradi, A.; Belouettar, S. Composite modeling for the effective elastic properties of semicrystalline polymers. J. Mech. Mater. Struct. 2007, 2, 1–21. [Google Scholar]
- Gueguen, O.; Ahzi, S.; Makradi, A.; Belouettar, S. A new three-phase model to estimate the effective elastic properties of semi-crystalline polymers: Application to PET. Mech. Mater. 2010, 42, 1–10. [Google Scholar]
- Anoukou, K.; Zaïri, F.; Naït-Abdelaziz, M.; Zaoui, A.; Qu, Z.; Gloaguen, J.M.; Lefebvre, J.M. A micromechanical model taking into account the contribution of α- and γ-crystalline phases in the stiffening of polyamide 6-clay nanocomposites: A closed-formulation including the crystal symmetry. Compos. Part B Eng. 2014, 64, 84–96. [Google Scholar]
- Yao, S.; Hu, D.; Xi, Z.; Liu, T.; Xu, Z.; Zhao, L. Effect of crystallization on tensile mechanical properties of PET foam: Experiment and model prediction. Polym. Test. 2020, 90, 106649. [Google Scholar]
- Bedoui, F.; Guigon, M. Linear viscoelastic behavior of poly(ethylene terephtalate) above Tg amorphous viscoelastic properties Vs crystallinity: Experimental and micromechanical modeling. Polymer 2010, 51, 5229–5235. [Google Scholar]
- Hachour, K.; Zaïri, F.; Naït-Abdelaziz, M.; Gloaguen, J.M.; Aberkane, M.; Lefebvre, J.M. Experiments and modeling of high-crystalline polyethylene yielding under different stress states. Int. J. Plast. 2014, 54, 1–18. [Google Scholar]
- Mesbah, A.; Elmeguenni, M.; Yan, Z.; Zaïri, F.; Ding, N.; Gloaguen, J.M. How stress triaxiality affects cavitation damage in high-density polyethylene: Experiments and constitutive modeling. Polym. Test. 2021, 100, 107248. [Google Scholar]
- Liu, H.T.; Sun, L.Z. Multi-scale modeling of elastoplastic deformation and strengthening mechanisms in aluminium-based amorphous nanocomposites. Acta Mater. 2005, 53, 2693–2701. [Google Scholar]
- Ju, J.W.; Sun, L.Z. Effective elastoplastic behavior of metal matrix composites containing randomly located aligned spheroidal inhomogeneities. Part I: Micromechanics-based formulation. Int. J. Solids Struct. 2001, 38, 183–201. [Google Scholar]
- Simo, J.C.; Kennedy, J.G.; Govindjee, S. Non-smooth multisurface plasticity and viscoplasticity. Loading/unloading conditions and numerical algorithms. Int. J. Numer. Methods Eng. 1988, 26, 2161–2185. [Google Scholar]
- Ju, J.W.; Zhang, X.D. Effective elastoplastic behavior of ductile matrix composites containing randomly located aligned circular fibers. Int. J. Solids Struct. 2001, 38, 4045–4069. [Google Scholar]
- Salem, D.R. Development of crystalline order during hot-drawing of poly(ethylene terephthalate) film: Influence of strain rate. Polymer 1992, 33, 3182–3188. [Google Scholar]
- Yan, Z.; Guo, Q.; Zaïri, F.; Zaoui, A.; Jiang, Q.; Liu, X. Continuum-based modeling large-strain plastic deformation of semi-crystalline polyethylene systems: Implication of texturing and amorphicity. Mech. Mater. 2021, 162, 104060. [Google Scholar]
- Basiri, A.; Zaïri, F.; Azadi, M.; Ghasemi-Ghalebahman, A. Micromechanical constitutive modeling of tensile and cyclic behaviors of nano-clay reinforced metal matrix nanocomposites. Mech. Mater. 2022, 168, 104280. [Google Scholar]






| Parameter | Significance | Value |
|---|---|---|
| Modulus | 118 GPa | |
| Poisson’s ratio | 0.3 | |
| Aspect ratio | 2 | |
| Reference strain rate | 2.1/s | |
| Maximum crystal degree | 0.3 | |
| Avrami exponent | 3 | |
| Number density of nuclei | 108 |
| Parameter | Significance | Value |
|---|---|---|
| Glassy modulus | 2.1 GPa | |
| Rubbery modulus | 18 MPa | |
| Glass transition temperature | 77 °C | |
| Temperature shift | 10 °C | |
| Transition slope | −0.04 MPa/°C | |
| Glassy Poisson’s ratio | 0.32 | |
| Rubbery Poisson’s ratio | 0.49 | |
| Initial yield strength | 3 MPa | |
| Hardening | 7 MPa | |
| Hardening | 0.9 | |
| Viscosity | 0.01 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mamache, F.E.; Mesbah, A.; Bian, H.; Zaïri, F. Micromechanical Modeling of the Biaxial Deformation-Induced Phase Transformation in Polyethylene Terephthalate. Polymers 2022, 14, 3028. https://doi.org/10.3390/polym14153028
Mamache FE, Mesbah A, Bian H, Zaïri F. Micromechanical Modeling of the Biaxial Deformation-Induced Phase Transformation in Polyethylene Terephthalate. Polymers. 2022; 14(15):3028. https://doi.org/10.3390/polym14153028
Chicago/Turabian StyleMamache, Fateh Enouar, Amar Mesbah, Hanbing Bian, and Fahmi Zaïri. 2022. "Micromechanical Modeling of the Biaxial Deformation-Induced Phase Transformation in Polyethylene Terephthalate" Polymers 14, no. 15: 3028. https://doi.org/10.3390/polym14153028